Time lapse video of painting game 2

Time lapse video of painting game 5

Max Tohline
2017
algorithm, lottery tickets, tape, adhesive
as installed: 45x66 in

What’s the half-life of money spent on lottery tickets? To find out, I devised a simple algorithmic project.

Procedure
Step 1. Purchase lottery tickets and hang them on a wall. (For this project, I purchased 50 tickets for the June 12, 2017 drawing of the Missouri Lottery’s Lucky for Life game: five each from ten different gas stations, supermarkets, and liquor stores in Rolla, MO. By buying from ten different stores, I ensured color and design variation in the paper they were printed on, adding some slight visual interest.)
Step 2. After the drawing, cash in any that win.
Step 3. Return to Step 1 until no tickets win and the project ends.

Research
Conceptual artists have made stochastic works involving money before (cf. Les Levine’s work, as recorded here in Lucy Lippard’s 6 Years), but I haven’t encountered one where the lottery is the mechanic and the rate of loss is the specific object of study. According to the Missouri Lottery’s website, the chances of winning any prize on Lucky for Life is 1 in 7.8. If one were to purchase an unlimited number of tickets, such that one could expect to win all the possible prizes at statistically-expected rates, the expected value of the $2 ticket would be $1.15. Since Lucky for Life drawings happen twice weekly, the half-life of money dumped into an unlimited number of tickets would be 4.375 days (assuming instantaneous lump-sum payouts immediately “re-invested”). But if you were to buy a small number of tickets (like I did), such that it would only be reasonable to anticipate winning some combination of the three lowest-value prizes (their odds of winning are given as 1/15, 1/32, and 1/50), the expected value of a $2 ticket drops to just 44 cents. That corresponds to a half-life of 1.614 days, which is the half-life expected for this project.

Results
Of the 50 tickets purchased, 6 won a prize. Of the 9 tickets purchased with that prize money, only 1 won a prize, which furnished money to buy 2 more tickets, both of which lost. 50 tickets became 9 tickets in half a week, then 9 tickets became 2 tickets in another half-week. That works out to an average half life of 1.521 days, or a little less than the hypothetical half-life suggested by the odds given on the Missouri Lottery’s website.

Discussion
In hindsight, I think I did this project to disgust myself with how quickly I could piss away a hundred bucks playing the lottery. But in the back of my mind, I harbored this irrational hope that buying 50 tickets at once actually made it likely that I might win a big prize. I fantasized about having to abandon the project halfway through because I hit the jackpot. Of course that didn’t happen; of course I wasted the money. But the fantasy is powerful and hard to shake, despite having a math minor and even after doing this project. Whenever I stop for gas, I still want to drop a few bucks on lotto tickets, even though I know what an irrational, wasteful decision that is.
Call it the flip side of the law of large numbers: if a large enough sample size of randomly-behaving operators (molecules of a gas, radioactive atoms, or lottery tickets, take your pick) behaves perfectly predictably in the aggregate, then it’s reasonable to expect that a small sample size will behave unpredictably. That doesn’t mean it’s likely to win (it’s not), but for some reason it makes it easier for us to believe… that it might? It’s like the mother who (irrationally) thinks her child might be president or a star athlete someday but (rationally) looks at all their classmates and knows none of them will make it. I think that most of us understand at least some aspect of the law of large numbers, we just have trouble placing ourselves within it.
What’s more, celebrity culture inundates us with survivorship bias. By flooding us with images of the wealthy and successful, media lead us to overestimate our own chances of success. We even see it in the natural world. For billions of years, death has sifted unfit organisms and species so well that now the biosphere teems with incredibly well-evolved creatures. On the one hand, the plenitude of life may trick us into thinking that life is easy, or inevitable. On the other hand, the apparent design of it all may beguile us into assuming the presence of a creator. We only see the living, none of the innumerable dead, and we draw misguided conclusions. Half-Life inverts that selection bias by showing only the dead.

Visit any antique mall and you’ll find thousands of old cans, bottles, glasses, mugs, and other containers. But despite the fact that these solid vessels all once held some sort of liquid, now they are nearly all empty and dry. It got me thinking about the cultural associations of permanence and transience which we ascribe to solids and liquids, respectively. So I looked for as many cans and bottles as I could find which still had some liquid in them and were, as such, in a sense still “alive” to their purpose as containers, not yet voided carcasses, and I gathered them here.

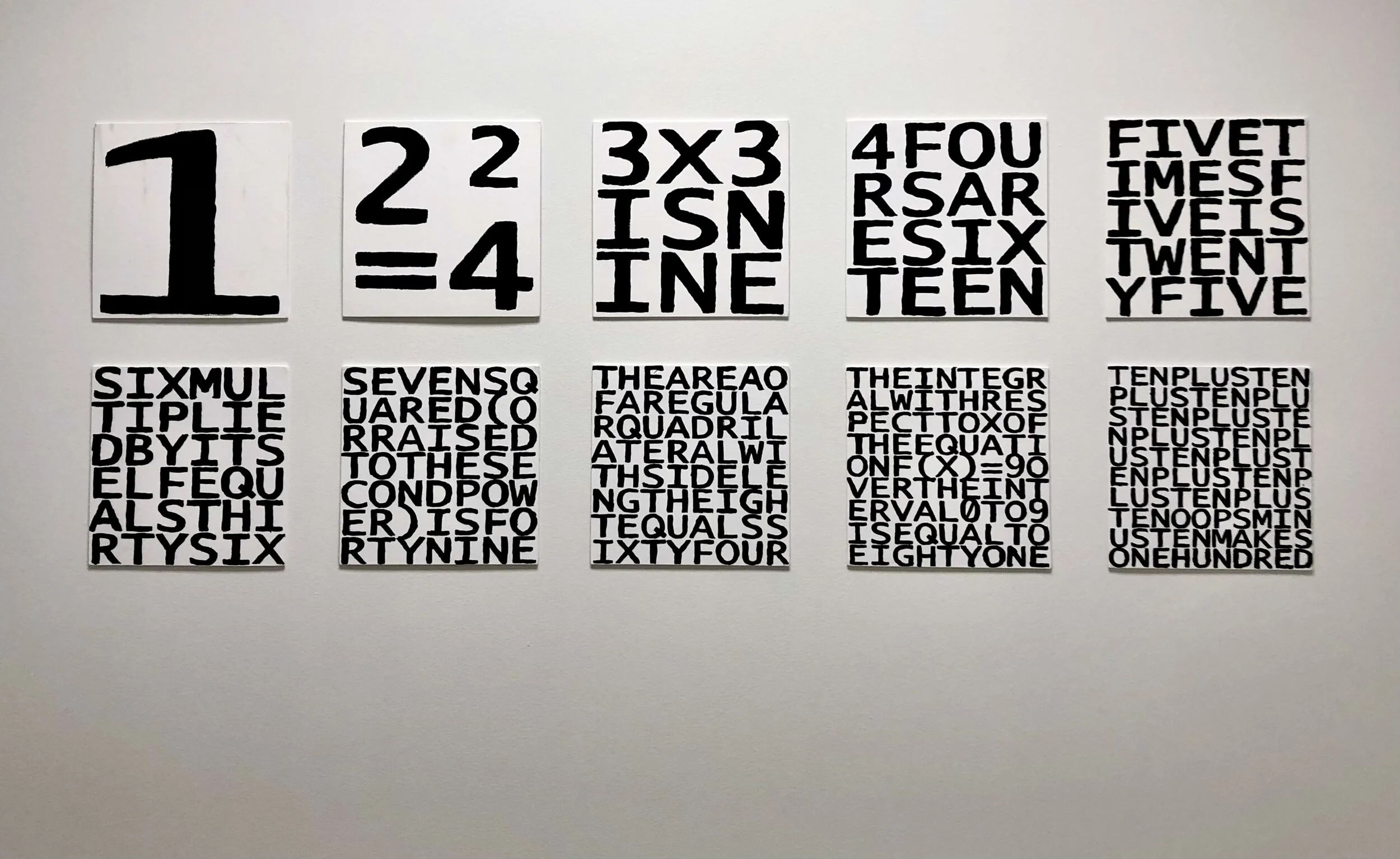
[Title Withheld] is a kind of a dreamcatcher fashioned out of K’Nex and populated with Scrabble tiles. It takes my love of words and my love of design back to their genetic roots and proposes a playful but complex synthesis of the two.

While the piece functions well enough as a purely aesthetic object, for adventurous viewers it may also be approached as a maze-like puzzle. The rules and goal of the puzzle are explained in detail on the image below, but in essence the point is to spell words which begin and end with the letter A by moving through the puzzle in a particular way.

I know of at least 20 more solutions to this puzzle. Some are names of places, which means that [Title Withheld] is a landscape; some are names of buildings or institutions, which means that [Title Withheld] reflexively refers to its own architecture as a maze, and the word “Areola” even shows up in there, which I guess means that [Title Withheld] is also a nude.

The real title of [Title Withheld] is the longest solution to the puzzle.

In July 2010, I was visiting the National Gallery of Art in Washington, DC, when I discovered this card in place of a painting in one of the galleries. As I leaned in to read it, I realized that my face and posture probably looked no different from anyone else admiring an artwork, except that I was no longer looking at something which was intended to be regarded as an artwork. Tickled by this, I decided that as a joke I should turn this card into an artwork. So I plucked it off the wall while no one was looking, tucked it into a museum map, smuggled it out, and later framed and titled it. Voilà!– an artwork.

Art is What is On the Wall comments on the fact that in the post-Brillo-Box era, it’s impossible to tell (based on any set of purely aesthetic criteria) what is or is not an artwork. Anything could be promoted to the status of art simply by being placed inside an institution – the museum or gallery – which confers the status of “art” onto the objects therein. BUT, here, on the wall where art was meant to be hanging, in the very spot reserved for a 15-Century French painting, was an object which is NOT art. But why isn’t it art? Aesthetics? Intent? Context? Deixis?

At the time, I taught introductory art classes, and my students often had trouble nailing down a definition of art which encompassed everything that we call art these days. In frustration, sometimes a student would just tautologically define art as “anything that has been called art.” Which is another way of saying, “if it’s on the wall of the art museum, it’s art.” But here was an object on the wall of an art museum (like those water fountains and exit signs in Andrea Fraser’s Museum Highlights) which was NOT art. Perhaps just to make a joke, or perhaps to force the resolution of an apparent paradox, I felt the need to turn this card into an artwork. So here it is. Art is What is On the Wall.

I spent my 2007 spring break installing this mathematical mural in the 89.7FM KMNR studios in Altman Hall, Missouri S&T (then UMR). Though the numbers on this mural may at first seem randomly arranged, their order is dictated by a simple arithmetic algorithm. In its installed location, the viewer is given no clues about this, as I wished to put the reasoning and interaction of the spectator under examination.

Though the algorithm used goes by many names, its most common name is the Collatz Algorithm, and it specifies the following rule: if a number is odd, multiply it by three and add one. If a number is even, divide by two. It is conjectured that if this process is repeated long enough, any natural number will eventually converge to one. I created the mural by beginning at one and working the calculation backwards. When a number exceeded 5,000, I removed it from the mural, noting its (dis)appearance with an asterisk. To streamline this manual computation process, I found it useful to highlight multiples of three in yellow on my studies, an aesthetic choice that carries over to the completed mural.

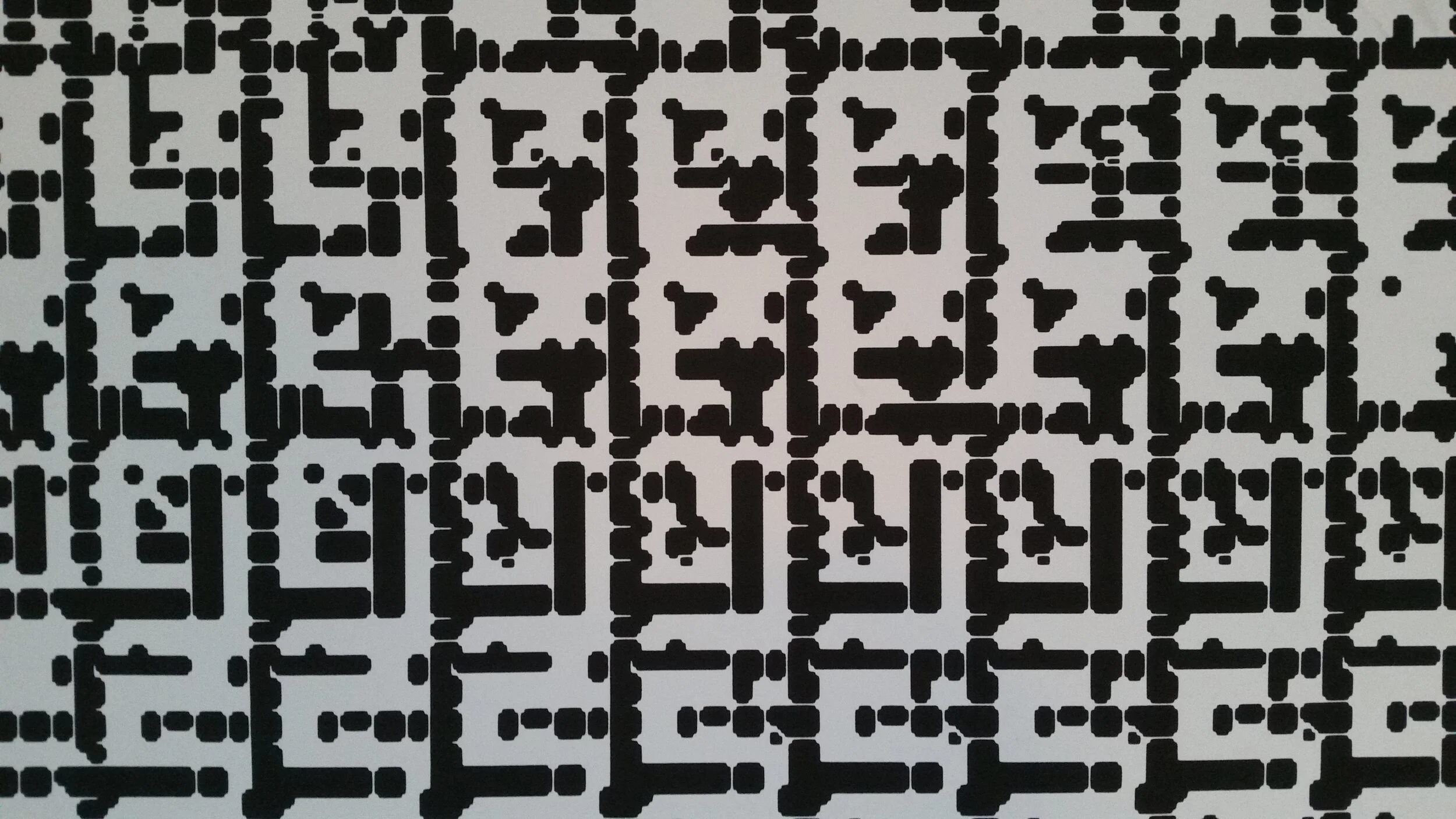
At least two mistakes appear on Converging to One. But there’s a happy accident as well: 897, which is ten times KMNR’s frequency [89.7] appears near the very end. I didn’t plan this. It just happened.

installation images and collage of preparatory studies courtesy of Ron Erickson